To know, “what is scientific notation in physics?” is very important for a student of science. It is also known as standard form of a number or simply standard form.
Table of Contents
Introduction
Understanding scientific notation is fundamental concept in the field of science, particularly in fields like chemistry, physics, astronomy, geography, and biology, where dealing with extremely large or tiny numbers is routine.
Also known as the “standard form,” scientific notation simplifies how we write and interpret these values. From measuring the vast distances between stars to the tiniest unseen-able particles, scientific notation provides a universal tool for expressing numbers compactly and clearly.
What is Scientific Notation?
It can be defined as, “a method used to express very large or very small numbers in a compact form.”
This approach is particularly useful in fields like science, engineering, and mathematics, where extremely large or tiny numbers are often used.
For example, the normal form of a number, say 5,000,000,000, is difficult to write. It is because, it can take up a lot of space. Using standard form, we can simplify it to 5 × 10⁹, which is much easier to handle.
Similarly, for very small numbers like 0.0000007, we can express it as 7 × 10⁻⁷.
Why is Scientific Notation Important?
Standard form of a number plays an important role in simplifying calculations involving extremely large or small values. Without scientific notation, it would be much harder to read and work with such numbers.
Whether we are measuring the distance between stars or the size of microscopic particles, this scientific form allows us to work with numbers without needing to write out every single zero.
For example:
- In Astronomy, scientists use this notation to express the distance between planets and galaxies. A distance like 5,878,499,810,000 kilometers (the distance light travels in a year) can be written as 5.88 × 10¹² kilometers.
- In Biology, scientists use it to measure microscopic organisms, where a bacteria size of 0.000001 meters can be written as 1 × 10⁻⁶ meters.
How to Write Numbers in Scientific Notation?
To write a number in standard notation, follow these steps:
Move the Decimal Point
The first step is to move the decimal either to the right or to the left in the number (depending upon the situation), so that only one non-zero digit is remained to the left of the decimal point.
Count How Many Places
After the decimal point has been moved, count the number of time it was move. This will be the exponent (power) of 10.
- If we move the decimal point to the left, the exponent will be positive.
- If we move the decimal point to the right, the exponent will be negative.
Using Prefixes with Scientific Notation
To make measurements even simpler, standard notation is often combined with prefixes. These prefixes, like kilo (k), milli (m), and micro (µ), represent powers of ten and help express large or small numbers in a more concise way.
Here is how scientific form/notation and prefixes work together:
- 1 kilometer (km) is equal to 1 × 10³ meters.
- 5 millimeters (mm) is equal to 5 × 10⁻³ meters.
- 2 megameters (Mm) is equal to 2 × 10⁶ meters.
This system is especially useful in fields like engineering, where distances and sizes can vary greatly.
Examples
A couple of examples related to standard form of a number are discussed here. (Note, it is assumed here that the reader (YOU) already has the knowledge about basic mathematical concepts like “powers and roots.”)
A Large Number
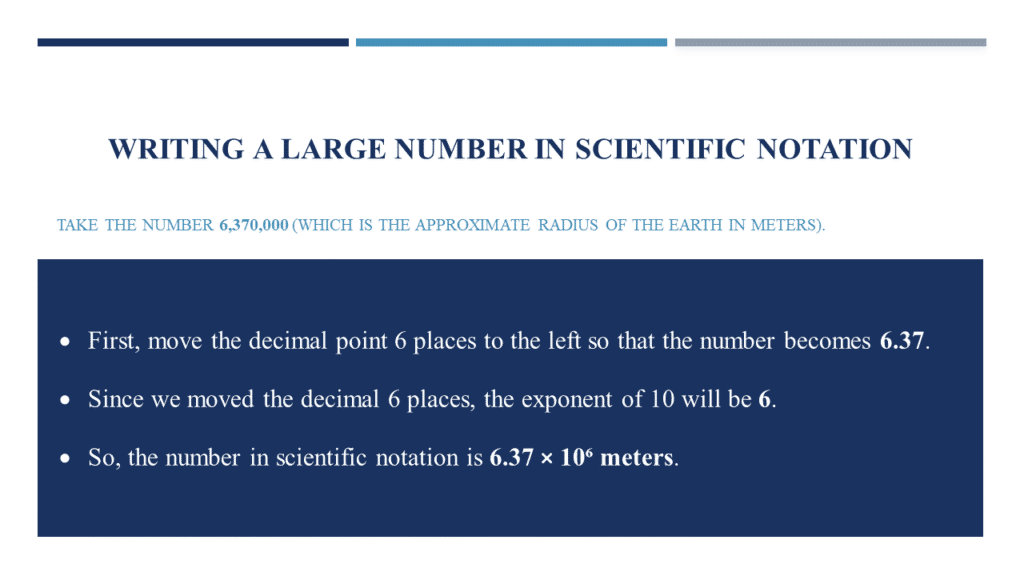
A Small Number
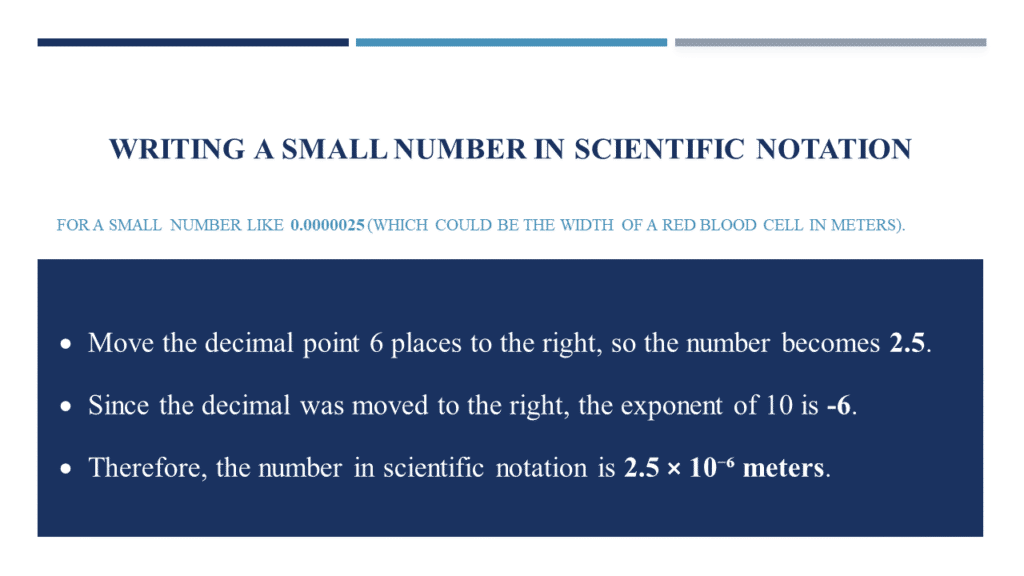
Number with Prefix
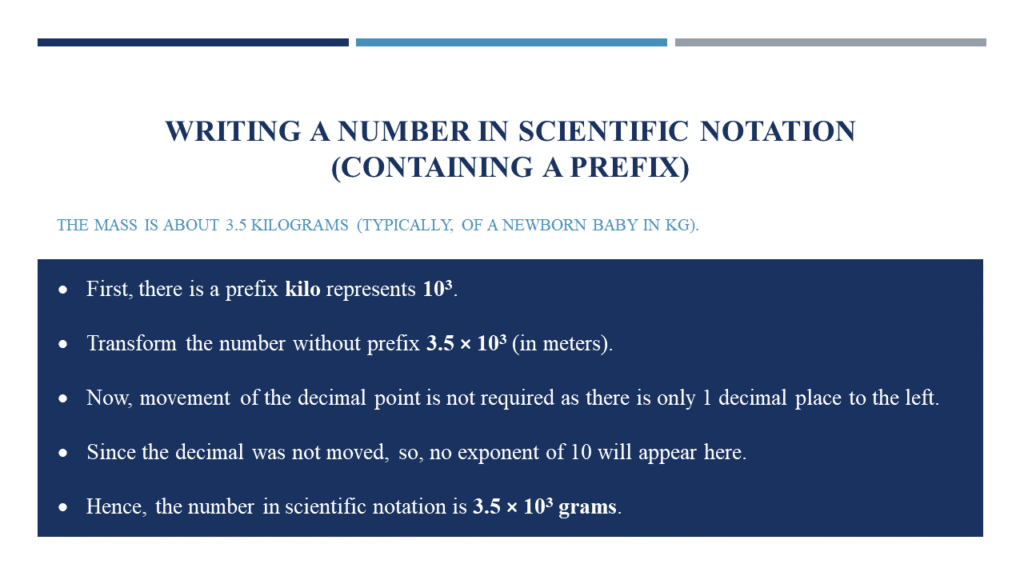
Number with Prefix and Exponent
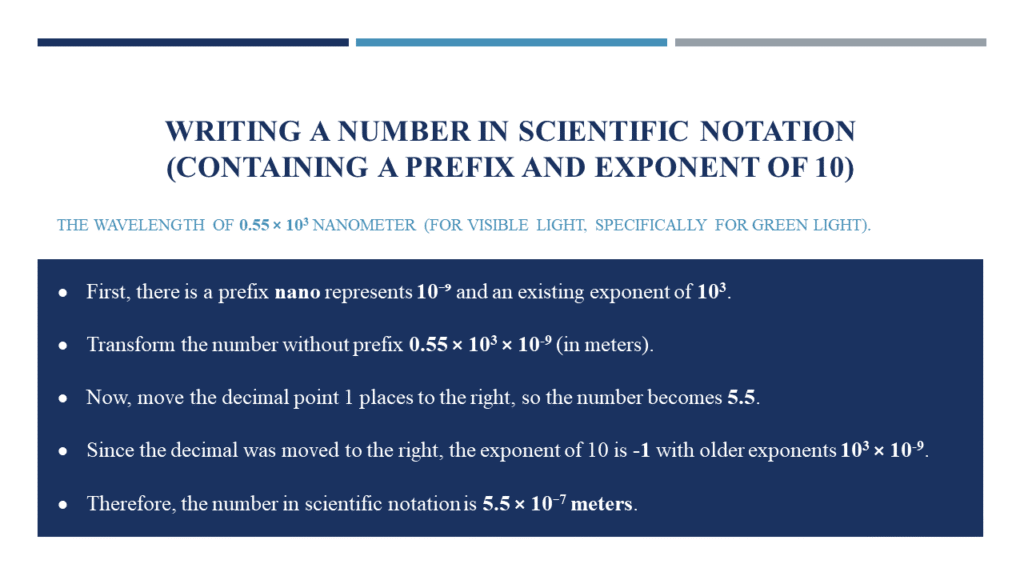
Real-Life Applications of Scientific Notation
Standard notation is not just used in classrooms. It is widely used in various fields, including:
Astronomy
Astronomers often work with very large distances, such as the distance between stars or galaxies. For example, the distance from Earth to the Sun is about 149,600,000 kilometers, which can be written as 1.496 × 10⁸ kilometers.
Physics
Physicists deal with both extremely large and extremely small measurements. For instance, the mass of a hydrogen atom is 0.00000000000000000000000000167 kilograms, which is much easier to write as 1.67 × 10⁻²⁷ kilograms.
Biology
Biologists often measure microscopic objects, such as cells or bacteria, using scientific notation. The size of a typical bacterial cell, for instance, is around 0.000001 meters, which is written as 1 × 10⁻⁶ meters.
Conclusion
Scientific form/notation is a powerful tool for simplifying calculations with very large or very small numbers. It allows us to manage complex data in a more efficient way, whether in science, engineering, or everyday life.
By understanding this system and learning how to use it alongside metric prefixes, we can easily navigate through the vast and tiniest scales of the universe. Whether a student or a professional, scientific notation enhances clarity and precision in both calculations and communication.
Frequently Asked Questions (FAQs)
What is scientific notation, and why is it used?
It is a way to express very large or very small numbers using powers of ten. It is used to simplify numbers and make calculations easier, especially when dealing with extremely big or tiny values.
How do I convert a number into scientific notation?
To convert a number into this notation, move the decimal point until there is only one non-zero digit to the left. Then, count how many places you moved the decimal to determine the power of 10.
What is the difference between positive and negative exponents in scientific notation?
A positive exponent means the number is large, while a negative exponent means the number is small.
Can I use scientific notation in everyday life?
Yes! The notation is not just for scientists. It can be used in everyday life for simplifying big numbers, like distances between cities, or small numbers, like the thickness of a sheet of paper.
What are the real-life applications of scientific notation?
Scientific notation is used in various fields, including astronomy (to measure distances in space), physics (to measure atomic sizes), and biology (to measure microscopic organisms).
How do I multiply or divide numbers in scientific notation?
When multiplying numbers in scientific notation, multiply the coefficients (the numbers before the 10) and add the exponents. When dividing, divide the coefficients and subtract the exponents.
Can scientific notation be used with prefixes?
Yes, scientific notation can be combined with metric prefixes like kilo (10³), milli (10⁻³), and micro (10⁻⁶) to express measurements more easily.
