A comparison of distance vs displacement in physics reveals that these two terms carry two different meanings in science in contrast to the literature. Discover the key differences between distance and displacement in physics.
Table of Contents
Introduction
In physics, understanding motion is crucial, and two important terms that often come up are distance and displacement. While they both describe how far something moves, their meanings and how we calculate them are different.
Understanding these concepts is essential for studying motion and solving real-world problems in physics. Learning the difference between the two is good for having strong foundation in kinematics and kinetics (in short, dynamics).
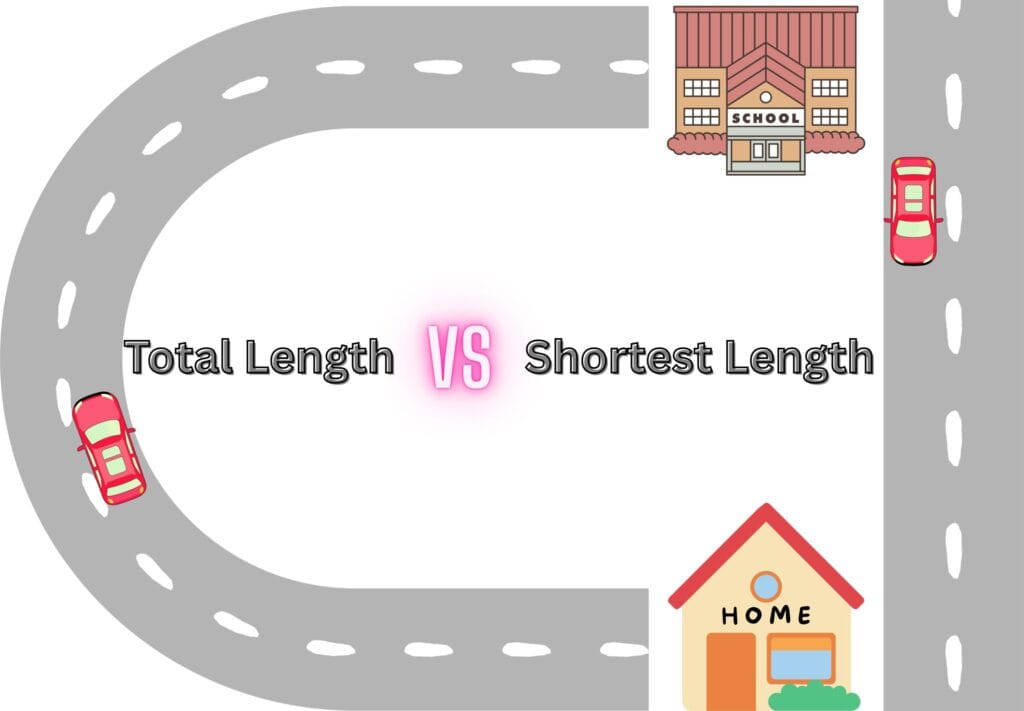
Distance
It is defined as,
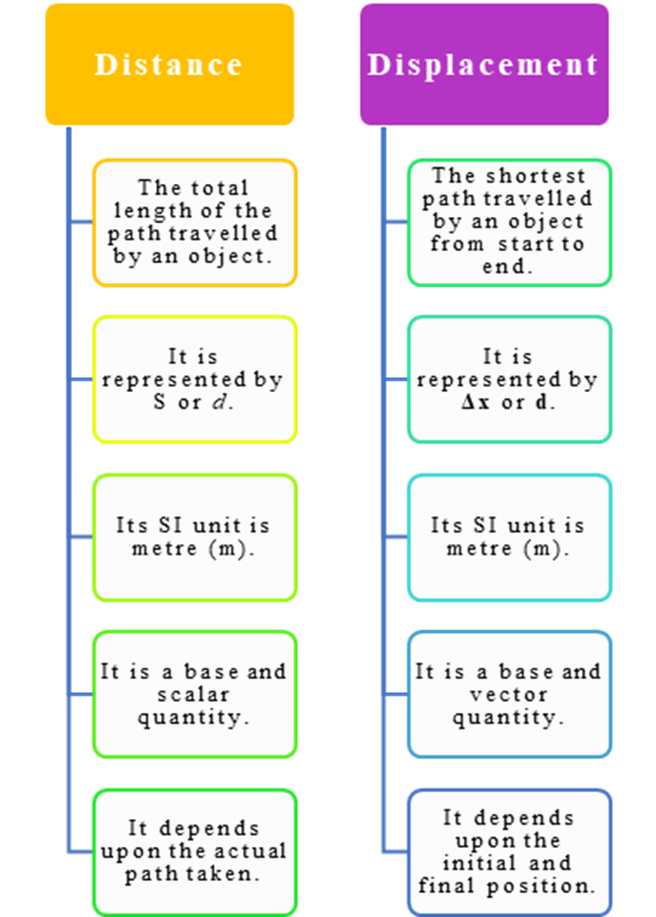
“the total length of the path travelled by an object, regardless of the direction.”
It tells us how much ground an object has covered or walked during its motion. Whether we walk in a straight line, circle, or zigzag, distance adds up all the steps that we take.
Symbol
The symbol used to represent it is usually S or d.
Type of Quantity
Similar to length, it is a base quantity in physics because it is directly measured in the form of length.
Unit
In the International System of Units (SI), it is measured in meters (m).
Nature of Quantity
It is a scalar quantity because it only requires magnitude (how much), and there is no need for direction.
Mathematical Formulation
For a straight path, the total length between two points is simply the absolute difference between the starting and ending positions. It is given as,
d = ∣x1 − x0∣
Where,
- x0 is the starting position.
- x1 is the ending position.
However, determining the total length becomes more complex if the path is not straight.
In such cases, the total length is the sum of all the small segments along the path. This can be achieved using calculus, more specifically by integration. In this process, infinitesimally small patches over the curve are summed up to get the total length travelled.
Displacement
It is defined as,
“the shortest straight-line distance travelled by an object from initial position to its final position, considering the direction.”
It tells us how far and in which direction an object has moved from its starting point.
An important characteristic of this quantity is that if an object completes a back-and-forth journey (meaning, returns to its initial position), then its magnitude will be zero. It is because, the final position coincides with the starting point.
Symbol
It is represented by Δx or simply d.
Type of Quantity
It is also a base quantity in physics, as it represents a form of length.
Unit
Similar to total length, it is measured in meters (m) in the SI system.
Nature of Quantity
It is a vector quantity. This means it has both magnitude and direction.
Mathematical Formulation
For a straight path (i.e., in 1–D), it is determined using the formula,
d = x1 – x0
Where,
- x0 is the starting position.
- x1 is the ending position.
For motion in 2–D or 3–D, it is calculated using the Pythagorean Theorem,
d = √[(x1 – x0)2 + (y1 − y0)2]
d = √[(x1 – x0)2 + (y1 − y0)2 + (z1 – z0)2]
This gives the straight-line length between the starting and ending points in 2–D or 3–D space.
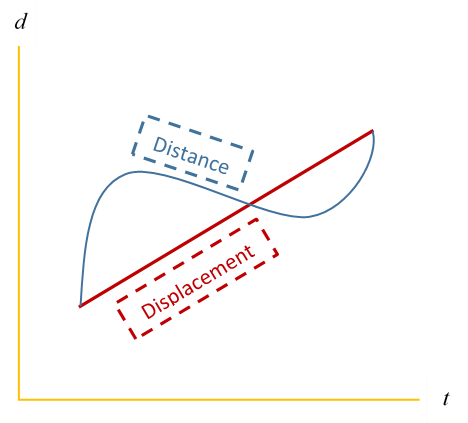
A Comparison of Distance vs Displacement in Physics
Conclusion
In summary, both distance and displacement measures of how far an object moves, but they have different meanings and uses.
One is a scalar quantity that measures the total path travelled, while the other is a vector quantity that measures the shortest path between the starting and ending points, including direction.
Although both are base quantities, displacement provides a more precise description of motion of an object in relation to its starting point.
Frequently Asked Questions (FAQs)
What is the difference between distance and displacement in physics?
The former refers to the total path traveled by an object, while the later is the shortest distance between the starting and ending points, including direction.
How do you calculate distance in physics for non-straight paths?
For non-straight paths, it is calculated by summing all the small segments along the path, often using calculus and integration for more complex paths.
What is the SI unit of distance and displacement?
According to the International System of Units (SI), both distance and displacement are measured in meters (m).
Why is displacement zero if an object returns to its starting point?
Actually, it measures the shortest straight length between two points. So, if the object returns to the starting point, it will become zero because the final and initial positions are the same.
How is displacement different from distance in terms of direction?
Distance and displacement are slightly different from each other. The former is a vector quantity and requires both magnitude and direction, whereas, the later is a scalar and requires only the magnitude for its complete description.
Can displacement be greater than distance?
No, a comparison of distance and displacement shows that it cannot be greater. It is because, displacement measures the shortest path, while the other considers the entire path travelled.
What is the mathematical formula to calculate displacement in 2-D space?
In 2-D space, it is calculated using the Pythagorean Theorem:
d = √[(x₁ – x₀)² + (y₁ – y₀)²].
How distance travelled is related to speed and time?
Mathematically, it is the product of speed and time and is given by the formula,
Distance = Speed × Time.
Why distance is considered a scalar quantity in physics?
It is considered a scalar quantity because it only measures magnitude, without any consideration of direction.
How can I easily remember the difference between distance and displacement?
Remembering the difference becomes easier if we know the difference between definitions of distance and displacement. The former measures the total journey, like counting steps, while the later is like drawing a straight line from start to end, accounting for direction.