In physics, the key difference between speed vs velocity lies in their definitions and nature of quantity.
Table of Contents
Introduction
When it comes to understanding the motion, speed and velocity are two fundamental concepts in physics. Although often used interchangeably in everyday conversation, these terms have distinct meanings in science.
Both terms are related to how fast an object is moving. However, they differ in the information they provide about the motion.
Speed
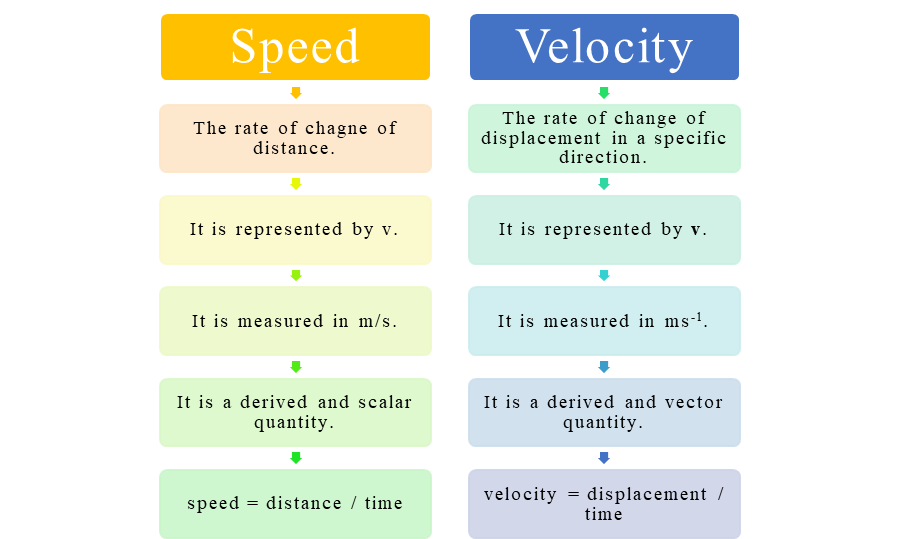
It is defined as,
“the rate of change of distance over a certain period of time.”
It tells us how fast an object is moving but does not provide any information about the direction of motion.
Symbol
The symbol usually used to represent it is ‘v’.
Type of Quantity
Speed is a derived quantity since it is calculated using two other physical quantities: distance and time.
Unit
The SI unit of speed is metres per second (m/s or ms-1).
Other units, like kilometres per hour (km/h), miles per hour (mph), knots, etc. are also commonly used.
Nature of Quantity
Speed is a scalar quantity. This means it only has magnitude and no direction.
Mathematical Formulation
Speed is calculated using the formula,
![]()
![]()
Velocity
It is defined as,
“the rate of change of displacement over time, in a specific direction.”
Unlike speed, velocity includes both magnitude and direction, making it a more detailed description of motion.
Symbol
It is commonly represented by the symbol v.
Type of Quantity
It is also a derived quantity, as it depends on displacement and time.
Unit
The SI unit of velocity is metres per second (m/s or ms-1), the same as speed. However, velocity includes directional information, making it distinct.
Nature of Quantity
It is a vector quantity. This means it has both magnitude and direction.
Mathematical Formulation
It is given by the formula,
![]()
![]()
Difference between Speed vs Velocity
Constant Velocity vs Variable Velocity
Knowing the difference between constant velocity vs variable velocity is quite important as it helps us in problem solving.
Constant Velocity (Uniform Motion)
When an object covers equal distances in equal intervals of time, without any change in its magnitude or direction, it is said to be in uniform motion with constant velocity.
Example
For example, a train moving at a steady speed of 80 km/h on a straight track is in uniform motion.
Variable Velocity (Non-Uniform Motion)
When an object covers equal distances in unequal intervals of time or unequal distance in equal interval of time, with either a change in its magnitude or direction, or both, it is said to be in non-uniform motion with variable velocity.
Example
For example, a car speeding up or slowing down while driving on a road experiences non-uniform motion. Similarly, a car moving on a circular track with same speed also undergoes variable velocity (although its speed is uniform).
Average Speed
It is defined as,
“the total distance travelled by an object divided by the total time taken to travel that distance.”
Average speed is calculated over a specified time interval. It gives an overall idea of the motion of an object from start to the end point.
Mathematical Formulation
Mathematically, it is represented as,
![]()
![]()
![]()
Example
For instance, if a cyclist travels a distance of 300 metres in 15 seconds, the average is,
![]()
Similarly, if a cyclist travel with a speed of 15 m/s for 10 seconds and then with a speed of 20 m/s for next 20 second, the average speed is,
![]()
Instantaneous Speed
It is defined as,
“the velocity of an object at a specific moment in time.”
It reflects how fast an object is moving at that particular instant, rather than over a period of time. Physically, it is measured using a speedometer in vehicles. Mathematically, it can be determined through calculus using the derivative of the position function with respect to time.
Mathematical Formulation
Mathematically, it is represented as follows,
![]()
Conclusion
Speed and velocity are both critical concepts in understanding motion in physics. While speed gives you an idea of how fast an object is moving, velocity goes a step further by including the direction of that movement.
These two quantities play a major role in everything from daily activities like driving to advanced fields like aerospace and engineering. Understanding their differences helps clarify the science behind motion, making it easier to predict, measure, and describe the movement of objects
Furthermore, concepts like uniform and non-uniform motion, as well as average and instantaneous speed and velocity, deepen our understanding of how objects behave in different scenarios.
Frequently Asked Questions (FAQs)
What is the key difference between speed and velocity in physics?
The main difference between speed and velocity lies in their definitions; speed is a scalar quantity representing how fast an object moves, while velocity is a vector quantity that includes both the speed of an object and its direction of motion.
How do average speed and instantaneous speed differ from each other?
Average speed is calculated over a specified time interval as the total distance divided by the total time taken, whereas instantaneous speed refers to the speed of an object at a specific moment in time, which can be measured using a speedometer.
What are examples of constant velocity and variable velocity in real life?
An example of constant velocity is a train moving at a steady speed on a straight track, while a car accelerating or decelerating on a road demonstrates variable velocity due to changes in speed and direction.
How can I mathematically calculate average speed for a journey?
To calculate average speed, divide the total distance traveled by the total time taken for the journey. For example, if a cyclist covers 300 meters in 15 seconds, the average speed would be,
vavg= 300 / 15 = 20 m/s
What are the implications of speed being a scalar quantity in physics?
As a scalar quantity, speed only indicates how fast an object is moving without any reference to direction. This means that knowing the speed alone does not provide full information about the motion of an object, which is crucial in scenarios where direction matters.
Can you explain the concepts of uniform and non-uniform motion?
Uniform motion occurs when an object travels equal distances in equal intervals of time at a constant speed, while non-uniform motion involves changes in speed or direction, resulting in unequal distances in equal time intervals.
What role does velocity play in kinematics and motion analysis?
Velocity is essential in kinematics because it provides detailed information about both the speed and direction of an object’s motion. This information is crucial for understanding the dynamics of moving objects and predicting their future positions.
How is instantaneous speed calculated in physics?
Instantaneous speed can be calculated using calculus, specifically through the derivative of the position function with respect to time. Mathematically, it is expressed as,
vins = lim ∆t → 0 (∆x / ∆t)
Why is it important to distinguish between speed and velocity in scientific discussions?
Distinguishing between speed and velocity is vital because it impacts how motion is described and understood. Misunderstanding these terms can lead to incorrect assumptions about the behavior and movement patterns of an object.
What units are commonly used to measure speed and velocity?
Both speed and velocity are measured in similar units, typically meters per second (m/s) in the SI system. Other units include kilometers per hour (km/h), miles per hour (mph), and knots, depending on the context of the measurement.