Power and root in mathematics are two essential concept after arithmetic operations of whole numbers, decimal numerals, fraction, and integers. In this blog, we shall explore these 2 concepts and their properties in detail.

Introduction

Understanding powers and roots is essential in mathematics, whether it is simplifying calculations or exploring algebraic expressions. Each concept, explain key insight of mathematical world, and their properties help solidify our understanding of mathematics.

What is Power?

In mathematics, the term power refers to the expression of the form aⁿ, where:

a is the base.
n is the exponent (or power–a misnomer, also called as index in some regions).

This notation represents the base a is being multiplied by itself n times.

Definition of Power

The power of a number is simply the number of times a base number is multiplied by itself.

For example:

$a^2$ (read as ‘a’ raised to the exponent 2) means $a \times a$
$a^4$ (read as ‘a’ raised to the exponent 4) means $a \times a \times a \times a$ .

Types of Bases and Exponents (Index)

Numeric Base

If the base is a specific number, like aⁿ (or a^x) then a is called a numeric base.

Variable Base

If the base is a variable, like xⁿ (or x^x), then x is called a variable base.

Numeric Index

When the index is a number, like aⁿ (or xⁿ), then n is a numeric index.

Variable Index

If the index is a variable, like a^x (or x^x), then x is called a variable index (it usually appears in the outline of a high school student).

What is a Root?

A root is an expression of the form $\sqrt[n]{a}$ or $a^{\frac{1}{n}}$ , where:

$\sqrt{\phantom{}}$ represents the radical sign.
n is the index, or degree, of the root.
a is the radicand.

For example:

The square root of ‘a‘, denoted by $\sqrt{a}$ is $a^{\frac{1}{2}}$
The cube root of a, denoted by $\sqrt[3]{a}$ , is $a^\frac{1}{3}$ .

Features of Roots

The index n in $\sqrt[n]{a}$ is always positive. In the form of $\quad \phantom{}^{\frac{1}{n}}$ , it can be negative.
For square roots, the radical sign is written as $\sqrt{\phantom{}}\quad$ and for other roots, it is written as $\sqrt[n]{\phantom{}}$ (where n $\geq 3$ ).
The number of digits (n) in the square root of a given number can be determined by taking half of the total digits $\frac{n}{2}$ when n is even. If n is odd, it can be calculated as one more than half, expressed as $\frac{n + 1}{2}$ .

Properties of Power and Root in Mathematics

Their important properties are given below:

Properties of Power

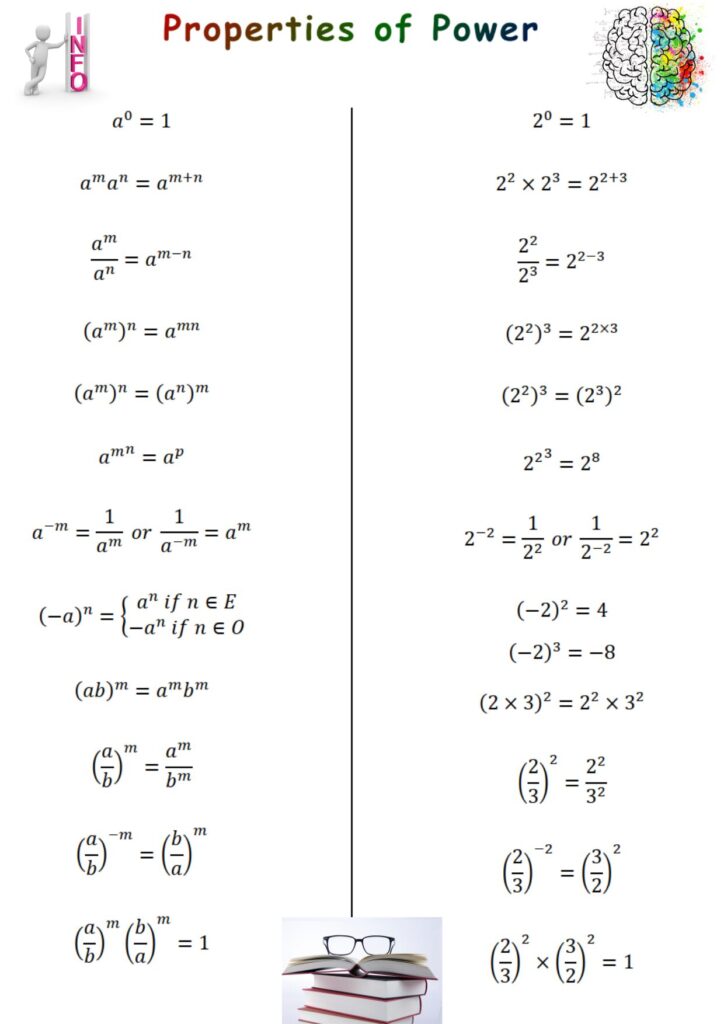
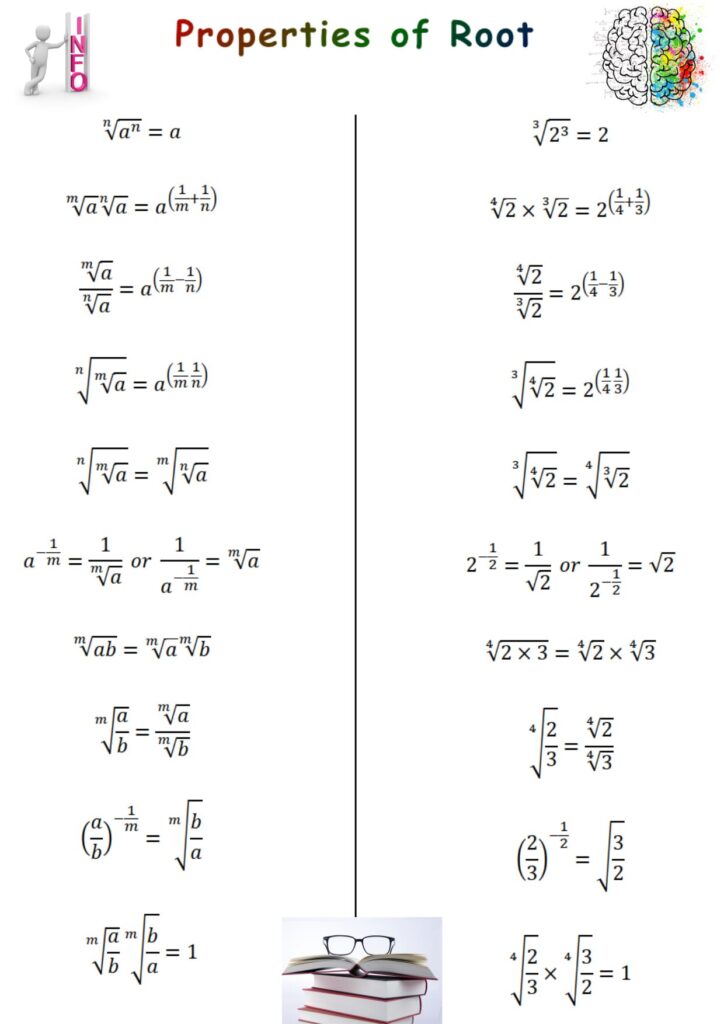
Properties of Root

Methods for Finding Powers and Roots

Powers

Patterns: Observing regular multiplication patterns.
Index Notation: Writing repeated multiplication in exponential form.
Mental Math: Applying known squares and cubes to simplify quickly.

Roots

Prime Factorisation Method: Breaking down numbers into prime factors to simplify square and cube roots.
Long Division Method: Useful for finding square roots of large numbers.
Approximation: Estimating roots, especially for non-perfect squares and cubes.

Key Takeaways of Power and Roots in Mathematics

Inverses

They are multiplicative inverses of each other. For example, squaring and taking the square root are opposites.

Sign of Powers

Even powers of negative numbers result in positive values (e.g., (−2)² = 4).
Odd powers of negative numbers remain negative (e.g., (−2)³ = −8).

Square Roots of Negatives

Square roots of negative numbers (like $\sqrt{-a}$ ) are complex numbers, and normally, is a part of high school student course outline.

Radicals and Zero

For fractions, the denominator of a radical expression cannot be zero.

Conclusion

Understanding powers and roots can make handling complex calculations simpler and unlock greater proficiency in algebra and calculus. Familiarising yourself with these rules and practicing examples will strengthen your ability to work with these foundational concepts in mathematics.

Frequently Asked Questions (FAQs)

What is a power in mathematics?

It represents a number raised to an exponent, showing repeated multiplication of the base by itself.

What does the index in a power term mean?

It tells how many times the base is multiplied by itself.

How do you read a power like a³?

a³ is read as “a raised to the power of 3” or simply “a cubed.”

What are common types of powers?

Common powers include squares (exponent of 2) and cubes (exponent of 3).

What is a root in mathematics?

It represents a number that, when multiplied by itself a certain number of times, gives the original value. For example, the square root of 9 is 3.

What is the difference between square and cube roots?

The square root finds a number that, when squared, equals the original, while the cube root finds a number that, when cubed, equals the original.

What is the relation between powers and roots?

They are inverses of each other; for instance, squaring and taking a square root are opposite operations.

Can you take the square root of a negative number?

Yes, but it results in a complex number, as the square root of a negative number is not real.

What methods can simplify calculating square roots?

Methods include prime factorization, long division, and approximation.

How are powers used in real-life applications?

These are used in calculating areas, volumes, scientific notation, and complex mathematical modeling.

Ali Abdullah

31 October 2024 at 5:35 PM

The blog post effectively explains powers and roots, including their definitions, properties, and calculation methods. It provides clear examples and insights, making it a valuable resource for understanding these fundamental mathematical concepts.

admin
1 November 2024 at 4:11 PM

Thank you for the kind response and appreciation. Feel free to explore more.

Power and Root in Mathematics | 2 Important Topics | Middle School and Beyond

Table of Contents

Introduction

What is Power?

Definition of Power